top of page

Review and Collinearity 

  • Quick review on the four centres' properties

  • Practice about the location of four centres

  • Discover the collinearity of centres

co.png

Review on Four Centres

Classification

Circumcentre

Height

Orthocentre

Not to Circle

Centroid

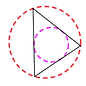
Incentre

Bisector

Not Circle_edited.jpg
Circumcentre.png
Orthocentre.png
Incentre.png
Height.png
Bisector.png

Relates

Centroid.png

--3 Points
   Cricle

--Tangent
   to Cricle

--Section     Formula

--Angle in
   semi-circle

To Circle

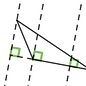
Relates to Height:

​

Centre can either be
"inside" - - - - - acute angle triangle
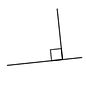
"On" - - - - - - - right angle triangle
"Outside" - - - - obtuse angle triangle
the triangle
Outside.png

Relates to Bisector:

​

Centre can only be
"inside" the triangle
Inside.png

Collinearity

雄蜂鏡頭

Discovery Zone

Collinear Points are the points that lie on the same straight line or in a single line

Characteristic:

In Four Centres we leant, only Centroid, Orthocentre and Circumcentre are collinear.

Incentre is not guarantee to be collinear with other centres 

But Why?
Explain by Venn Diagram

Bisect Line

Centroid

Circumcentre

Orthocentre

Bisect Angle

Incentre

Right Angle

Consolidation& Self-Evaluation

Get familiar on distinguishing

the location of four triangle centres

書籍堆疊
Read the statement discribing the triangle centres,
decides whether it is TRUE or FALSE

Capstone Project:

Learning Package for Triangle Centers
-5E instructional model

bottom of page